Question

A set of final examination grades in an introductory statistics course is normally​ distributed, with a mean of 77 and a standard deviation of 7. Complete parts​ (a) through​ (d). a. What is the probability that a student scored below 86 on this​ exam? The probability that a student scored below 86 is nothing. ​(Round to four decimal places as​ needed.)

Answer 1

0.9007 is the probability that a student scored below 86 on this​ exam.

Explanation:

We are given the following information in the question:

Mean, μ = 77

Standard Deviation, σ = 7

We are given that the distribution of examination grades is a bell shaped distribution that is a normal distribution.

Formula:

`z_(score) = \displaystyle(x-\mu)/(\sigma)`

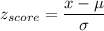
a) P(student scored below 86)

P(x < 86)

`P( x < 86) = P( z < \displaystyle(86 - 77)/(7)) = P(z < 1.2857)`

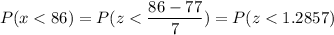
Calculation the value from standard normal z table, we have,

`P(x < 1.2857) = 0.9007 = 90.07\%`

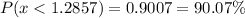
0.9007 is the probability that a student scored below 86 on this​ exam.