Question

In the exercise, X is a binomial variable with n = 9 and p = 0.3. Compute the given probability. Check your answer using technology. HINT [See Example 2.] (Round your answer to five decimal places.) P(X = 5)

Answer 1

Answer: The required probability is 0.07351.

Step-by-step explanation: Given that X is a binomial variable with n = 9 and p = 0.3.

We are given to compute the probability P(X = 5) and round the answer to five decimal places.

We know that

the binomial distribution formula for P(X = r) with n number of trials is given by

`P(X=r)=^nC_rp^rq^(n-r),~~\textup{where }q=1-p.`

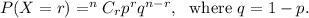
According to the given information, we have

n = 9, p = 0.3 and q = 1 - p = 1 - 0.3 = 0.7.

Therefore, we get

`P(X=5)\\\\=^9C_5(0.3)^5(0.7)^(9-5)\\\\\\=(9!)/(5!(9-5)!)(0.3)^5(0.7)^4\\\\\\=(9*8*7*6*5!)/(5!*4*3*2*1)*0.00273*.2401\\\\=126*0.000583443\\\\=0.073513818.`

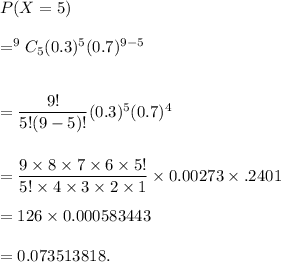
Rounding to five decimal places, we get

P(X=5) = 0.07351.

Thus, the required probability is 0.07351.