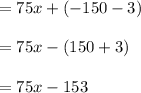Question

1. Solve.

The product of −2c+14 and the multiplicative inverse of −12


2. Solve.

10+(m−10)


3. Solve.

6w+3−3


4. Write the expression in standard form

13(3x+6)


5. Write the expression in standard form

15(5x−10)−3

Answer 1

1.
`(c)/(6)-(7)/(6)`

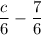
2. m

3. 6w

4. 39x + 78

5. 75x - 153

Explanation:

1. The product of −2c+14 and the multiplicative inverse of −12 is

`(-2c+14)\cdot (1)/(-12)`

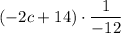
Use distributive property:

`(-2c+14)\cdot (1)/(-12)\\ \\=-(1)/(12)\cdot (-2c)-(1)/(12)\cdot 14\\ \\=(c)/(6)-(7)/(6)`

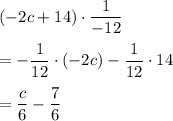
2. Solve 10+(m−10)

Open the brackets remembering that the sign before brackets is "+":

`10+(m-10)\\ \\=10+m-10`

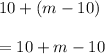
Combine the like terms usin commutative property:

`10+m-10\\ \\=(10-10)+m\\ \\=0+m \\ \\=m`

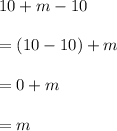
3. Solve 6w+3−3

Combine the like terms:

`6w+3-3\\ \\=6w+(3-3)\\ \\=6w+0\\ \\=6w`

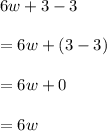
4. Write the expression in standard form 13(3x+6).

Use distributive property:

`13(3x+6)\\ \\=13\cdot 3x+13\cdot 6\\ \\=39x+78`

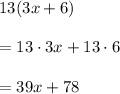
5. Write the expression in standard form 15(5x−10)−3.

Use distributive property:

`15(5x-10)-3\\ \\=15\cdot 5x-15\cdot 10-3\\ \\=75x-150-3`

Combine the like terms:

`=75x+(-150-3)\\ \\=75x-(150+3)\\ \\=75x-153`