Answer:
a)
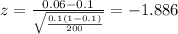
b) The 90% confidence interval would be given by (0.0324;0.0875)
So the confidence interval not contains the 0.1.
Explanation:
1) Data given and notation n
n=200 represent the random sample taken
X=12 represent the number of M&M's green
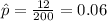 estimated proportion of M&M's green.
estimated proportion of M&M's green.
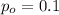 is the value that we want to test
is the value that we want to test
 represent the significance level
represent the significance level
z would represent the statistic (variable of interest)
 represent the p value (variable of interest)
represent the p value (variable of interest)
2) Concepts and formulas to use
We need to conduct a hypothesis in order to test the claim that the true proportion of M&M's green is 0.1. The system of hypothesis are:
Null hypothesis:
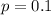
Alternative hypothesis:

When we conduct a proportion test we need to use the z statistic, and the is given by:
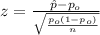 (1)
(1)
The One-Sample Proportion Test is used to assess whether a population proportion
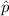 is significantly different from a hypothesized value
is significantly different from a hypothesized value
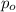 .
.
3) Calculate the statistic
Since we have all the info requires we can replace in formula (1) like this:
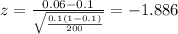
4) Statistical decision
P value method or p value approach . "This method consists on determining "likely" or "unlikely" by determining the probability assuming the null hypothesis were true of observing a more extreme test statistic in the direction of the alternative hypothesis than the one observed". Or in other words is just a method to have an statistical decision to fail to reject or reject the null hypothesis.
The significance level is not provided, but we can assume
 . The next step would be calculate the p value for this test.
. The next step would be calculate the p value for this test.
Since is a bilateral test the p value would be:
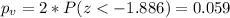
So based on the p value obtained and using the significance level assumed
 we have
we have
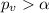 so we can conclude that we fail to reject the null hypothesis, and we can said that at 5% of significance the true proportion of M&M's green is not significantly different from 0.1 or 10% .
so we can conclude that we fail to reject the null hypothesis, and we can said that at 5% of significance the true proportion of M&M's green is not significantly different from 0.1 or 10% .
5) Confidence interval
In order to find the critical value we need to take in count that we are finding the interval for a proportion, so on this case we need to use the z distribution. Since our interval is at 90% of confidence, our significance level would be given by
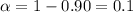 and
and
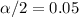 . And the critical values would be given by:
. And the critical values would be given by:

The confidence interval for the mean is given by the following formula:
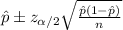
If we replace the values obtained we got:
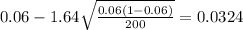
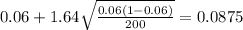
The 90% confidence interval would be given by (0.0324;0.0875)
So the confidence interval not contains the 0.1.