Question

A conical oil cup with A radius of 5.6cm must be design to hold 69 cu cm of oil. What should be the altitude of the cup

Answer 1

The height of the conical oil cup is 2.102 cm

Explanation:

Given as for a conical cup :

The radius of the conical oil cup = r = 5.6 cm

The volume of the conical oil cup = v = 69 cubic cm

Or, v = 69 cm³

Now, ∵ The volume of cone =
`(1)/(3)`×
`\pi`×r²×h

Where r is the radius of the cone

And h is the height of the cone

The value of
`\pi` = 3.14

So, The volume of conical oil cup = The volume of cone =
`(1)/(3)`×
`\pi`×r²×h

Or, The volume of conical oil cup =
`(1)/(3)` × 3. 14 × (5.6)² × h

Or, 69 cm³ =
`(1)/(3)` × 98.47 × h

Or, 69 cm³ = 32.823 cm² × h

∴ h =
`(69)/(32.823)`

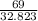
I,e h = 2.102 cm

So, height of cup is 2.102 cm

Hence The height of the conical oil cup is 2.102 cm , Answer