Question

A pharmacist has 40% and 60% iodine solutions on hand. How many liters of each item solution will be required to produce 8 L of a 50% iodine mixture

Answer 1

The quantity of 40 % solution is 4 liters and

The quantity of 60 % solution is 4 liters .

Explanation:

Given as

A pharmacist has 40% and 60% iodine solutions on hand.

Let the quantity of 40 % iodine solutions = x liter

And The quantity of 60 % iodine solutions = y liter

The total quantity of iodine mixture = 8 liter

I.e The quantity of 40 % iodine solutions + the quantity of 60 % iodine solutions = 8 Liter

or x + y = 8

The The percentage of mixture solution = 50 %

∴ 40 % of x + 60 % of y = 50 % of 8

I.e 40 x + 60 y = 400 ..........A

And x + y = 8

Or, 40× ( x + y ) = 40× 8

or, 40 x + 40 y = 320 ...........B

Now , solving equations A and B

I.e ( 40 x + 60 y ) - ( 40 x + 40 y ) = 400 - 320

Or, ( 40 x - 40 x ) + ( 60 y - 40 y ) = 80

Or, 0 + 20 y = 80

∴ y =
`(80)/(20)`

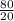
I.e y = 4 liters

So, The quantity of 60 % iodine solution = y = 4 liters

Put The value of y in Eq A

So , 40 x + 60 × 4 = 400

Or, 40 x = 400 - 240

or, 40 x = 160

∴ x =
`(160)/(40)`

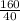
I.e x = 4 liter

So, The quantity of 40 % iodine solution = x = 4 liters

Hence The quantity of 40 % solution is 4 liters and the quantity of 60 % solution is 4 liters . Answer