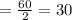Answer:30 ways
Explanation:
Given
there are total of 6 charms out of which 2 are same
total no of ways in which n person can be arranged in a round table is (n-1)!
So 6 charms can be arranged in a bracelet is (6-1)!
Now two charms are same i.e. total no of ways to arrange
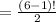
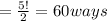
As rotation are indistinguishable i.e. clockwise become anti-clockwise therefore
total no of ways are