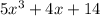Question

1. Part I: The degree of a polynomial is the (greatest / least) of the degrees of its terms. (Circle the term

that correctly completes this definition.) (1 point)

Part II: In order to write a polynomial in descending order, you must write the terms with the exponents

(decreasing / increasing) from left to right. (Circle the term that correctly completes this rule.) (1 point)

Part III: For each polynomial, determine the degree and write the polynomial in descending order.

(4 points: 2 points each)

A. -4x² - 12 + 11x

B. 2x3 + 14 - 3x + 7x+ 3x3

Answer 1

Part I: The degree of a polynomial is the greatest of the degrees of its terms.

Part II: In order to write a polynomial in descending order, you must write the terms with the exponents decreasing from left to right.

Part III: A. -4x² + 11x - 12, degree = 2,

B. 5x³ + 4x + 14, degree = 3.

Explanation:

Part I : Since, the degree of a polynomial is the highest power of its monomials ( single term ),

eg : degree of
`x^5 + 4x` is 5.

Thus, in part I, the correct option is 'greatest'

Part II : When we write a polynomial then we write the terms of the polynomial in descending order of their degrees.

Thus, in part II the correct option is 'least'

Part III : A.
`-4x^2 - 12 + 11x`

∵ -4x² has the highest power in the polynomial.

⇒ Degree = 2,

Also, in the polynomial descending order of degrees,

2 > 1 > 0

⇒ polynomial in descending order,

`-4x^2 + 11x - 12`

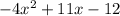
B.
`2x^3 + 14 - 3x + 7x + 3x^3`

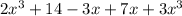
Combining like terms,

`5x^3 + 14+4x`

∵ 5x³ has the highest degree,

⇒ Degree = 3,

Also, the order of the degrees in the polynomial is,

3 < 2 < 1 < 0

Thus, the polynomial in descending order,

`5x^3+4x + 14`