Answer:
[Cd²⁺] = 5.82x10⁻³ M
[Mn²⁺] = 0.037M
Step-by-step explanation:
The titration of EDTA with Cd²⁺, Mn²⁺ and Ca²⁺ are based in the formation of complexes with a relation 1:1, EDTA: metal, so from the titration of the excess unreacted EDTA with Ca²⁺ we can calculate the EDTA moles by:
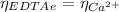
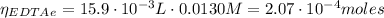
The moles of EDTA are:
 (1)
(1)
where
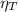 : is the total moles of EDTA,
: is the total moles of EDTA,
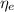 : is the EDTA excess moles and
: is the EDTA excess moles and
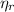 : is the EDTA moles that react with Cd²⁺ and Mn²⁺
: is the EDTA moles that react with Cd²⁺ and Mn²⁺
Hence the EDTA moles that react with Cd²⁺ and Mn²⁺ are equal to the moles of both metals:
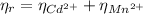 (2)
(2)
From equation (1) we can find the
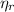 :
:
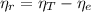

 (3)
(3)
Now, from the titration of the EDTA free of Cd²⁺ with Ca²⁺, we can calculate the moles of EDTA that react with the Cd²⁺ and hence the Cd²⁺ moles:


Having the Cd²⁺ moles we can now calculate the Mn²⁺ moles using equation (3):
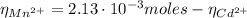

Now, with the Cd²⁺ moles and Mn²⁺ moles we can find their concentrations in the original solution:
![[Cd^(2+)] = (2.91 \cdot 10^(-4) moles)/(50.0\cdot 10^(-3)L) = 5.82 \cdot 10^(-3) M](https://img.qammunity.org/2020/formulas/chemistry/college/5hnm7sdneuh5ls2s6z3ayh8ofi5r4d173j.png)
![[Mn^(2+)] = (1.84 \cdot 10^(-3) moles)/(50.0\cdot 10^(-3)L) = 0.037M](https://img.qammunity.org/2020/formulas/chemistry/college/7pfcgptksr34ib9h4i5mzhy8c48zoqnajh.png)
I hope it helps you!