Answer:
N(1)=50 is a minimum
N(15)=4391.7 is a maximum
Explanation:
Extrema values of functions
If the first and second derivative of a function f exists, then f'(a)=0 will produce values for a called critical points. If a is a critical point and f''(a) is negative, then x=a is a local maximum, if f''(a) is positive, then x=a is a local minimum.
We are given a function (corrected)
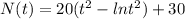
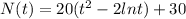
(a)
First, we take its derivative
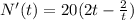
Solve N'(t)=0
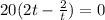
Simplifying
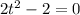
Solving for t
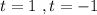
Only t=1 belongs to the valid interval
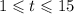
Taking the second derivative

Which is always positive, so t=1 is a minimum
(b)
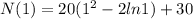
N(1)=50 is a minimum
(c) Since no local maximum can be found, we test for the endpoints. t=1 was already determined as a minimum, we take t=15
(d)
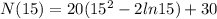
N(15)=4391.7 is a maximum