Question

A single crystal of a metal that has the BCC crystal structure is oriented such that a tensile stress is applied in the [010] direction. If the magnitude of this stress is 2.75 MPa, compute the resolved shear stress in the direction on each of the (110) and (101) planes. (b) On the basis of these resolved shear stress values, which slip system(s) is (are) most favorably oriented?

Answer 1

Thus the most favorable slip option is for [1 1 0] plane.

Solution:

As per the question:

Magnitude of the stress,
`\sigma = 2.75\ MPa`

No. of atoms in Body Centered Cubic Crystal, n = 2

Now,

Resolved shear stress is given by:

`\sigma_(R) = \sigma cos\theta cos\lambda`

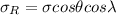
where

`\theta` = angle between slip plane and the tensile axis

`\lambda` = angle between tensile axis and the slip direction

Direction of applied force: [0 1 0]

Plane: [1 1 0]

Direction:
`[\bar{1}\ 1\ 1]`

Also, we know that:

`cos\theta = (a_(1)a_(2) + b_(1)b_(2) +c_(1)c_(2))/(|a||b|)`

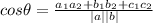
Thus

`cos\theta = \frac{(0* 1) + (1* 1) + (0* 0)}{1* \sqrt{1^(2) + 1^(2)} + {0}^(2)} = (1)/(√(2))`

`cos\lambda = \frac{(0* - 1) + (1* 1) + (0* 1)}{1* \sqrt{1^(2) + 1^(2) + 1^(2)}} = (1)/(√(3))`

`\sigma_(R) = \sigma cos\theta cos\lambda = 2.75* (1)/(√(2))* (1)/(√(3))`

`\sigma_(R) = 1.123\ MPa`

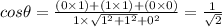
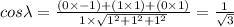
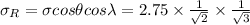
Now,

Direction of applied force: [0 1 0]

Plane: [1 0 1]

Direction:
`[\bar{1}\ 1\ 1]`

`cos\theta = \frac{(0* 1) + (1* 0) + (0* 1)}{1* \sqrt{1^(2) + 1^(2)}} = 0`

`cos\lambda = \frac{(0* - 1) + (1* 1) + (0* 1)}{1* \sqrt{1^(2) + 1^(2) + 1^(2)}} = (1)/(√(3))`

`\sigma_(R) = \sigma cos\theta cos\lambda = 2.75* 0* (1)/(√(3))`

`\sigma_(R) = 0 MPa`

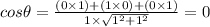
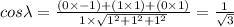
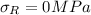
Now,

It is clear that for the first case, the resolved shear stress is higher.

Thus the most favorable slip option is for [1 1 0] plane.