Question

What is the solution to this system of equations?

-5.9x-3.7y=-2.1
5.9x+3.7y=2.1

Answer 1

the answer is C

Explanation:

Answer 2

There are infinite solutions that solve the system
`y=0.57-1.59x`

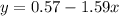
Explanation:

• The solution can be expressed as
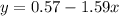  `y=0.57-1.59x` (there are infinite solutions that solve the system). This results from rearranging terms in the first or second equation.

• This is because the first equation is a linear combination of the second one (this is, the first equation equals (-1) times the second equation). This means that the second equation does not add any information about x and y: it says exactly the same as the first equation about the relationship between x and y.

• Then, in terms of solving the system, you have one unique expression (not repeated) that shows the relationship between y and x. This means that, any pair of (x,y) that meet the requirements expressed by
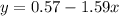  `y=0.57-1.59x`, will solve the system. Then we have infinite solutions.