Question

Arc QVT measures 156

Which statement must be true?

R

Angles QRT and QST both measure 78°

Angles QRT and QST both measure 156

Angle QRT measures 78° and angle QST measures

102

Angle QRT measures 156° and angle QST measures

24

Answer 1

A

Explanation:

e2020

Answer 2

Angles QRT and QST both measure 78 degrees

Explanation:

We are given that

Measure of arc QVT=
`156^(\circ)`

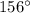
We know that

Central angle is equal to measure of intercepted arc

`arc QVT=\angle QPT=156^(\circ)`

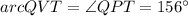
Inscribed angle formed by same arc are equal

`\angle QRT=\angle QST`

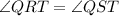
Inscribed angle is equal to half of the central angle.

`\angle QRT=\angle QST=(1)/(2)* 156=78^(\circ)`

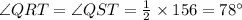
Hence, measures of angle QRT and angle QST are equal.

Therefore, option A is true.