Answer:
Explanation:
Given that there is a function of x,
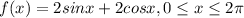
Let us find first and second derivative for f(x)
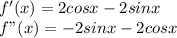
When f'(x) =0 we have tanx = 1 and hence
a) f'(x) >0 for I and III quadrant
Hence increasing in
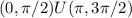
and decreasing in
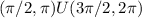
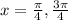
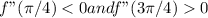
Hence f has a maxima at x = pi/4 and minima at x = 3pi/4
b) Maximum value =
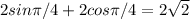
Minimum value =
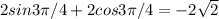
c)
f"(x) =0 gives tanx =-1
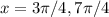
are points of inflection.
concave up in (3pi/4,7pi/4)
and concave down in (0,3pi/4)U(7pi/4,2pi)