Question

An object dropped from the top of the leaning tower of pisa lands 17 feet from the base

Answer 1

cos x=17/179

Explanation:

Answer 2

Cos x=
`(17)/(179)`

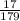
Explanation:

Here is the complete question: An object dropped from the top of the leaning tower of Pisa lands 17 feet from the base. Picture is attached.

Which equations should be used to determine the angle (x) that the leaning Tower of Pisa makes with the ground?

Given : The hypotenuse of the triangle formed by Pisa tower is 179 ft.

The Base or adjacent side of the triangle is 17 ft.

Now, using the cosine rule of trigonometry to know the angle x.

As we know, cosine
`\theta = (Adjacent)/(Hypotenuse)`

Cos(x)=
`(17)/(179)`

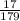
∴ The equation to determine the angle x is
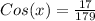`Cos (x)= (17)/(179)` that the leaning tower of pisa makes with the ground.