Question

from the top of the tower 30m hight a man who is 2m tall is observing the base of a tree at an angle of depression measuring 30 degree. Find the distance between the tree and the tower

Answer 1

The distance between the tree and the tower is 18.48 meters.

Explanation:

We are given the following information in the question:

Height of tower = 30 m

Height of man = 2 m

Angle of depression = 30 degrees

We have to find the distance between the tree and the tower.

The attached image shows the scenario.

Formula:

`\text{Tan(Angle of Depression)} = \displaystyle\frac{\text{Perpendicular}}{\text{Base}} = \frac{\text{Distance between tower and tree}}{\text{Height of tower + Height of man}}\\\\= \tan(30) = \frac{\text{Distance between tower and tree}}{32}\\\\(1)/(\sqrt3) = \frac{\text{Distance between tower and tree}}{32}\\\\\text{Distance between tower and tree} = (32)/(\sqrt3) = 18.48~m`

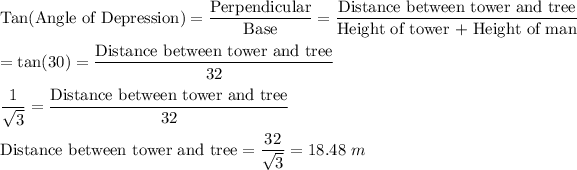
Thus, the distance between the tree and the tower is 18.48 meters.