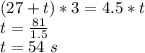Question

wanda started walking along a path 27 seconds before Dave .Wanda walked at a constant rate of 3 feet per second. Dave walked along the same path at a constant rate of 4.5 feet per second. how long after Dave starts walking will he catch up with Wanda.?

Answer 1

54 seconds

Explanation:

Wanda's walking rate = 3 ft/s

Wanda's head start = 27 s

Dave's walking rate= 4.5 ft/s

Let 't' be time after Dave started walking

The distance walked by Wanda and Dave, respectively, as a function of 't' are given by:

`W(t) = (27+t)*3\\D(t) = 4.5*t`

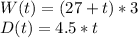
The instant 't' at which Dave catches up to Wanda (both distances walked are equal) is:

`(27+t)*3= 4.5*t\\t=(81)/(1.5) \\t= 54\ s`