Answer:
The number of tickets for students sold was 836 and the number of tickets for general admission sold was 1,121
Explanation:
The correct question is
Tickets for a high school football game cost $2 for students and $3 for general admission. 1,957 tickets were sold and $5,035 was collected. How many of each type of tickets was sold?
Let
x ----> number of tickets for students sold
y ----> number of tickets for general admission sold
we know that
 ---->
---->
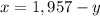 ----> equation A
----> equation A
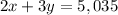 ----> equation B
----> equation B
Solve the system by substitution
substitute equation A in equation B
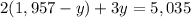
solve for y

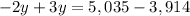
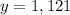
Find the value of x
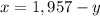 ---->
---->

therefore
The number of tickets for students sold was 836 and the number of tickets for general admission sold was 1,121