Question

The point P(2k, k) is equidistant from A(-2, 4) and B (7,-5).
Find the value of k.
​

Answer 1

k = 3

Explanation:

Using the distance formula

d = √ (x₂ - x₁ )² + (y₂ - y₁ )²

with (x₁, y₁ ) = A (- 2, 4 ) and (x₂, y₂ ) = P (2k, k)

AP =
`√((2k+2)^2+(k-4)^2)`

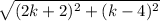
Repeat

with (x₁, y₁ ) = B (7, - 5) and P = (2k, k)

BP =
`√((2k-7)^2+(k+5)^2)`

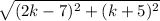
Given that AP = BP, then

`√((2k+2)^2+(k-4)^2)` =
`√((2k-7)^2+(k+5)^2)`

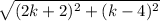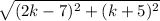
Square both sides

(2k + 2)² + (k - 4)² = (2k - 7)² + (k + 5)² ← expand factors on both sides

4k² + 8k + 4 + k² - 8k + 16 = 4k² - 28k + 49 + k² + 10k + 25

Simplify both sides by collecting like terms

5k² + 20 = 5k² - 18k + 74 ( subtract 5k² from both sides )

20 = - 18k + 74 ( subtract 74 from both sides )

- 54 = - 18k ( divide both sides by - 18 )

k = 3