Answer:
58 ounces of 32% solution and 29 ounces of 38% solution should be mixed.
Explanation:
Let, 87 ounces of a 34% solution is prepared by mixing x ounces of 32% alcohol solution and y ounces of 38% alcohol solution.
Hence, x + y = 87 .......... (1)
And,
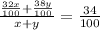
⇒

⇒ 0.32x + 0.38y = 29.58
⇒ x + 1.1875 y = 92.4375 ......... (2)
Now, from equations (1) and (2) we get
(1.1875 - 1)y = 92.4375 - 87
⇒ y = 29
Hence, from equation (1) we get, x = 87 - 29 = 58
Therefore, 58 ounces of 32% solution and 29 ounces of 38% solution should be mixed. (Answer)