Question

A stragitic defense strategy calls for missiles to be fired independelty at a target until the target us hit. Let the probability that any missile will hit the target be .8 and x=# of missiles fired at the target

Answer 1

Points:

The question is incomplete as the value of x is not given and what is required is not stated.

However, I'll assume that x = 5

Going by the details of the question, possible questions could be:

• Expected number of hits
• Variance and Standard Deviation of hits

Answer:

See Explanation

Explanation:

Given

Represent the probability with p.

So:

`p = 0.8`

Solving (a): The expected number of hits.

In probability, the expected number is calculated as:

`E(x) = p * x`

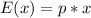
Substitute values for p and x

`E(x) = 0.8 * 5`

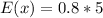
Remember that 5 is an assumed value of x

`E(x) = 4`

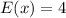
Hence, the expected number of hits when 5 missiles is fired is 4

Solving (b): Variance

In probability, variance is calculated as:

`variance = x*p*(1-p)`

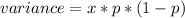
Substitute values for p and x

`variance = 5*0.8*(1-0.8)`

`variance = 5*0.8*0.2`

`variance = 0.8`

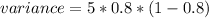
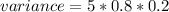
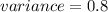
Hence, the variance is 0.8

Solving (c): The standard deviation

In probability, the standard deviation is calculated as:

`SD= \sqrt{Variance`

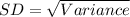
Substitute values for variance

`SD= \sqrt{0.8`

`SD= 0.89`

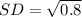
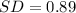
Hence, the standard deviation is 0.89