Question

You plan to work for 40 years and then retire using a 25-year annuity. You want to arrange a retirement income of $4500 per month. You have access to an account that pays an APR of 7.2% compounded monthly. What size nest egg do you need to achieve the desired monthly yield?

What montly deposits are required to achieve the desired monthly yield at retirement?

Answer 1

623,459.79 and 224.51

Step-by-step explanation:

first lets consider the first part of the problem and is how mucho do i need to accumulate for having an annuity for 25 years. this problem can be solved applying the concept of annuity, keep in mind that an annuity is a formula which allows you to calculate the present value of future payments affected by an interest rate.by definition the present value of an annuity is given by:

`a_(n) =P*(1-(1+i)^(-n) )/(i)`

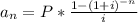
where
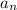`a_(n)` is the present value of the annuity,
`i` is the interest rate for every period payment, n is the number of payments, and P is the regular amount paid. so applying to this particular problem, we have:

`a_(25*12) =4,500*(1-(1+0.006)^(-25*12) )/(0.006)`

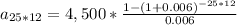
look at the value 25*12 because the problem tells us is during 25 years but the payment is monthly, and look at the 0.006 and it is comming from the APR/12 and we must do that because this rate is componded Monthly:

`a_(25*12) =623,459.79`

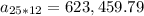
so for the second part we must calculate the second part we must calculate the acumulated value at 40 years of work:

`s_(n) =P*((1+i)^(n)-1 )/(i)`

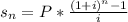
where
`s_(n)` is the future value of the annuity,
`i` is the interest rate for every period payment, n is the number of payments, and P is the regular amount paid. so applying to this particular problem, we have:

`623,459.79 =P*((1+0.006)^(40*12)-1 )/(0.006)`

solving for P we have:

P=224.51