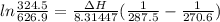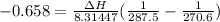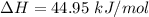Question

The vapor pressure of the liquid HF is measured at different temperatures. The following vapor pressure data are obtained: Temperature 270.6K and 287.5K, Pressure 324.5 mmHg and 626.9 mmHG. Calculate the enthapy of vaporization ( delta H vap ) in kJ/mol for this liquid.

Answer 1

Enthalpy is 44.95 kJ/mol

Solution:

As per the question:

Temperature, T = 270.6 K

Temperature, T' = 287.5 K

Pressure, P = 324.5 mmHg

Pressure, P' = 626.9 mmHg

Now,

To calculate the enthalpy, we make use of the Clausius-Clapeyron eqn:

`ln(P)/(P') = (\Delta H)/(R)((1)/(T') - (1)/(T))`

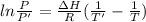
where

`\Delta H = Enthalpy`

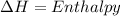
R = Rydberg's constant

Substituting suitable values in the above eqn:

`ln(324.5)/(626.9) = (\Delta H)/(8.31447)((1)/(287.5) - (1)/(270.6))`

`- 0.658 = (\Delta H)/(8.31447)((1)/(287.5) - (1)/(270.6))`

`\Delta H = 44.95\ kJ/mol`