Answer:
The carriage speed B was 67.4 mph
Step-by-step explanation:
This is an exercise for the moment, that as a vector we must look for the solution of each axis (x, y). We define a system formed by the two cars, for this system the forces during the crash are internal, so the moment is preserved.
The data they give is the car more A m = 1000kg and its speed is v1₁₀ = 30 mph i^ and the mass of the car B M = 1500 kg
Let's write the moment for each axis
X axis
p₀ₓ =
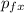
m v₁ₓ + 0 = (m + M) vₓ
Y axis
poy =
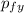
0 + M
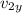 = (m + M)
= (m + M)
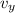
Let's look for the components of the final velocity with trigonometry
sin 66 =
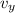 / v
/ v
cos 66 = vₓ / v
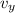 = v sin 66
= v sin 66
vₓ = v cos 66
We substitute and write the system of equations
m v₁ₓ = (m + M) v cos 66
M
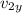 = (m + M) v sin66
= (m + M) v sin66
From the first equation
v = m / (m + M) v₁ₓ / cos 66
v = 1000 / (1000 + 1500) 30 / cos 66
v = 29.5 mph
From the second equation
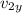 = (m + M)/m v sin 66
= (m + M)/m v sin 66
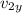 = (1000 + 1500) /1000 29.5 sin 66
= (1000 + 1500) /1000 29.5 sin 66
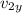 = 67.4 mph
= 67.4 mph
The carriage speed B was 67.4 mph