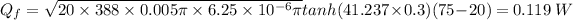Question

A straight fin is made from copper (k = 388 W/m-K) and is 0.5 cm in diameter and 30 cm long. The temperature at the base of the fin is 75oC and it is exposed to flowing air at 20oC with h = 20 W/m2-K. That is the rate of heat transfer from this pin?

Answer 1

The rate of transfer of heat is 0.119 W

Solution:

As per the question:

Diameter of the fin, D = 0.5 cm = 0.005 m

Length of the fin, l =30 cm = 0.3 m

Base temperature,
`T_(b) = 75^(\circ)C`

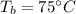
Air temperature,
`T_(infty) = 20^(\circ)`

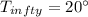
k = 388 W/mK

h =
`20\ W/m^(2)K`

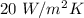
Now,

Perimeter of the fin, p =
`\pi D = 0.005\pi \ m`

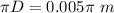
Cross-sectional area of the fin, A =
`(\pi)/(4)D^(2)`

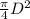
A =
`(\pi)/(4)(0.5* 10^(-2))^(2) = 6.25* 10^(- 6)\pi \ m^(2)`

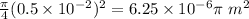
To calculate the heat transfer rate:

`Q_(f) = √(hkpA)tanh(ml)(T_(b) - T_(infty))`

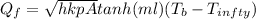
where

`m = \sqrt{(hp)/(kA)} = \sqrt{(20* 0.005\pi)/(388* 6.25* 10^(- 6)\pi)} = 41.237`

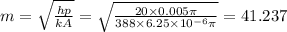
Now,

`Q_(f) = \sqrt{20* 388* 0.005\pi* 6.25* 10^(- 6)\pi}tanh(41.237* 0.3)(75 - 20) = 0.119\ W`