Answer: The population proportion is between 0.34 and 0.46 .
Explanation:
Interpretation of 95% confidence interval : A person can be about 95% confident that the true population parameter lies in the interval.
A confidence interval is constructed for an unknown population proportion, p. A sample is collected, and the 95% confidence interval is calculated to be 0.40 ± 0.06.
i.e. Lower limit =
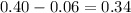
Upper limit =
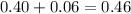
i.e. 95% confidence interval = (0.34, 0.46)
i.e. A person can be about 95% confident that the true population proportion (p) lies in the interval (0.34, 0.46).
⇒ It is most accurate to say that there is approximately 95% confidence in the assertion that:
The population proportion is between 0.34 and 0.46 .