Answer:
Remember, if
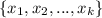 is a basis for a subspace W of
is a basis for a subspace W of
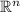 then
then
 is an orthonormal basis of W, where
is an orthonormal basis of W, where
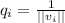 and
and
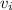 is defined as:
is defined as:
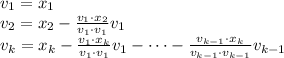
Then, to find a orthonormal basis of
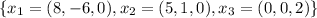 we will find first the
we will find first the
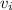 's.
's.

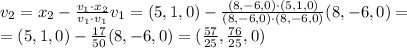
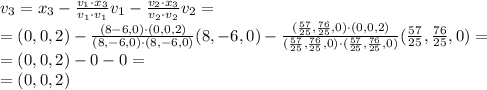
Therefore,
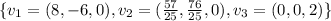 is a ortogonal basis for
is a ortogonal basis for
 . But we need a orthonormal basis. Then is enough find the corresponding unit vector of the ortogonal basis found.
. But we need a orthonormal basis. Then is enough find the corresponding unit vector of the ortogonal basis found.
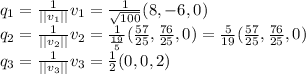
Hence
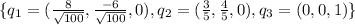 is a orthonormal basis for
is a orthonormal basis for
