Answer: c)

Explanation:
Given: Nestor Milk Powder is sold in packets with an advertised mean weight of 1.5 kgs.
i.e.
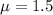
A consumer group wishes to check the accuracy of the advertised mean and takes a sample of 52 packets finding an average weight of 1.49 kgs.
So 1.49 is sample mean but hypothesis is the statement about the parameter which is
 .
.
i.e. he wanted to check whether
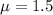 or
or

Since null hypothesis
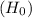 contains equality and alternative hypothesis
contains equality and alternative hypothesis
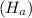 is against it.
is against it.
Therefore, the set of hypotheses that should be used to test the accuracy of advertised weight would be :

Hence, the correct answer is option c) oH: μ= 1.5; 1H: μ≠1.5