Answer : The solubility of
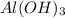 is
is

Explanation :
First we have to calculate the pOH.
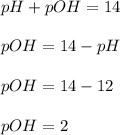
Now we have to calculate the concentration of
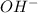 .
.
![pOH=-\log [OH^-]](https://img.qammunity.org/2020/formulas/chemistry/high-school/h1t4ubcsdqvqg0xpalkkvnwrun04y9pzd8.png)
![2=-\log [OH^-]](https://img.qammunity.org/2020/formulas/chemistry/high-school/u6vgs56kk1wf1cdivds65dwst5cqjyr1re.png)
![[OH^-]=0.01](https://img.qammunity.org/2020/formulas/chemistry/high-school/f8dxn0d7wgg5s8zoijpuo5sj0afb2kaygb.png)
The solubility equilibrium reaction will be:

The expression for solubility constant for this reaction will be,
![K_(sp)=[Al^(3+)][OH^(-)]^3](https://img.qammunity.org/2020/formulas/chemistry/high-school/vb400zn0mf7f4cmzm8s8hnbok8wpj2loau.png)
Now put all the given values in this expression, we get:
![1.0* 10^(-33)=[Al^(3+)]* (0.01)^3](https://img.qammunity.org/2020/formulas/chemistry/high-school/oc7wbpbbr52ri9i9wl4obhn8elxy0i963y.png)
![[Al^(3+)]=1.0* 10^(-27)M](https://img.qammunity.org/2020/formulas/chemistry/high-school/e4bu6eosz7b7oqyspb4l74a9ds5ya0suv4.png)
As, the solubility of
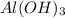 =
=
![[Al^(3+)]](https://img.qammunity.org/2020/formulas/chemistry/college/7alerx4hp12zkqu9rvclpa6xcpb2kmxn98.png) =
=

Thus, the solubility of
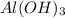 is
is
