Answer:
Induced EMF,
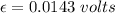
Step-by-step explanation:
Given that,
Radius of the circular loop, r = 5 cm = 0.05 m
Time, t = 0.0548 s
Initial magnetic field,
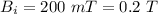
Final magnetic field,

The expression for the induced emf is given by :
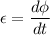
 = magnetic flux
= magnetic flux
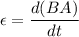
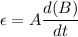
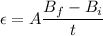
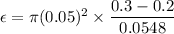
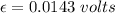
So, the induced emf in the loop is 0.0143 volts. Hence, this is the required solution.