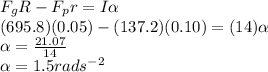Question

A high-wire walker always attempts to keep his center of mass (com) over the wire (or rope). He normally carries a long, heavy pole to help: If he leans, say, to his right (his com moves to the right) and is in danger of rotating around the wire, he moves the pole to his left (its com moves to the left) to slow the rotation and allow himself time to adjust his balance. Assume that the walker has a mass of 71.0 kg and a rotational inertia of 14.0 kg · m² about the wire. What is the magnitude of his angular acceleration about the wire if his com is 5.0 cm to the right of the wire under the following conditions?

(a) he carries no pole and
(b) the 14.0 kg pole he carries has its com 10 cm to the left of the wire?

Answer 1

a)

2.5 rads⁻²

b)

1.5 rads⁻²

Step-by-step explanation:

a)

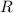`R` = distance of the center of mass from the wire = 5 cm = 0.05 m

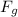`F_(g)` = Force of gravity on walker

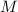`M` = mass of walker = 71 kg

Force of gravity on walker is given as

`F_(g) = Mg\\F_(g) = (71)(9.8)\\F_(g) = 695.8 N`

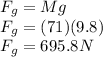
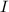`I` = Rotational inertia of walker = 14 kgm²

`\alpha` = Angular acceleration about the wire

Torque equation is given as

`F_(g) R = I \alpha \\(695.8) (0.05) = (14) \alpha \\\alpha = ((695.8) (0.05))/(14) \\\alpha= 2.5 rads^(-2)`

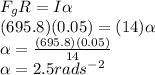
b)

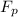`F_(p)` = Force of gravity on pole

`m` = mass of walker = 14 kg

Force of gravity on pole is given as

`F_(p) = mg\\F_(g) = (14)(9.8)\\F_(g) = 137.2 N`

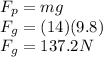
`r` = distance of the center of mass of pole from the wire = 10 cm = 0.10 m

Torque equation is given as

`F_(g) R - F_(p) r = I \alpha \\(695.8) (0.05) - (137.2)(0.10) = (14) \alpha \\\alpha= (21.07)/(14) \\\alpha=1.5 rads^(-2)`