Question

ABCE is a trapet AD is parallel to BC DE=4cm area of ABCE=60cm?area of ABCD=48cm work out the values of f and g show all your working please be quick thank youuuu

Answer 1

The value are

f = 6 cm

g = 8 cm

Explanation:

Given:

ABCE is Trapezium

AD || BC

AD = BC = f

CD = g

Area of ABCE = 60 cm²

Area of ABCD = 48 cm²

To Find :

f = ?

g = ?

Solution:

In the figure ∠ C = 90° and AD || BC

∴ ∠ D = 90° .....Corresponding angles are equal

∴ ABCD is RECTANGLE With Length = f and Breadth = g

∴
`\textrm{Area of Rectangle}=Length* Breadth\\\textrm{Area of Rectangle}=f* g\\`

`\textrm{Area of Triangle AED}= \textrm{Area of Trapezium ABCE}-\textrm{Area of Rectangle ABCD}\\=60-48\\=12\ cm^(2) \\\therefore \textrm{Area of Triangle AED} = (1)/(2)* AD* DE\\12 =(1)/(2)* f* 4\\ f=(24)/(4)\\\therefore f= 6\ cm`

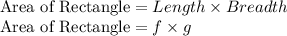
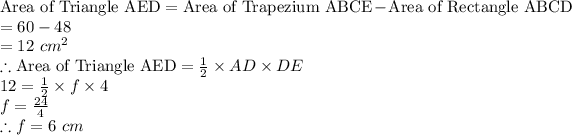
Now,

∴
`\textrm{Area of Rectangle}=Length* Breadth\\\textrm{Area of Rectangle}=f* g\\`

`\therefore 48 = f* g\\48= 6* g\\\therefore g =(48)/(6)\\ g=8\ cm`

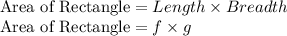
The value are

f = 6 cm

g = 8 cm