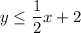Question

On a coordinate plane, a solid straight line has a positive slope and goes through (negative 4, 0) and (0, 2). Everything to the right of the line is shaded. Which linear inequality is represented by the graph? y ≤ One-halfx + 2 y ≥ One-halfx + 2 y ≤ One-thirdx + 2 y ≥ One-thirdx + 2

Answer 1

A

y ≤
`(1)/(2)`x + 2

Explanation:

Answer 2

`y\le(1)/(2)x+2`

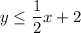
Explanation:

On a coordinate plane, a solid straight line has a positive slope and goes through (negative 4, 0) and (0, 2). This line has the equation

`y-0=(2-0)/(0-(-4))(x-(-4))\\ \\y=(1)/(2)(x+4)\\ \\y=(1)/(2)x+2`

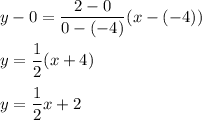
The origin belongs to the shaded region, so its coordinates must satisfy the inequality. Since

`(1)/(2)\cdot 0+2=2\ge 0,`

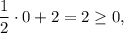
then the correct inequality is

`y\le(1)/(2)x+2`