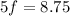Question

Melissa bought 3 loaves of freshly baked bread at a specialty bread shop. She paid twice as much for the whole grain bread as for the French bread, and $2.50 more for the cinnamon raisin bread than for the whole grain bread. She spent a total of $11.25 for the 3 loaves. If f represents the price of a loaf of French bread, which equations describe this situation? Select two answers

A.

f + f + (f + 2.50) = 11.25

B.

f + 2f + (f + 2.50) = 11.25

C.

f + 2f + (2f + 2.50) = 11.25

D.

2f + 2.50 = 11.25

E.

5f = 8.75

Answer 1

The correct equations that describe this situation are: A. f + f + (f + 2.50) = 11.25 and D. 2f + 2.50 = 11.25.

Step-by-step explanation:

The correct equations that describe this situation are:

1. f + f + (f + 2.50) = 11.25
2. 2f + 2.50 = 11.25

To solve this problem, let's assign variables to represent the prices. Let f be the price of a loaf of French bread. According to the information given, the whole grain bread costs twice as much as the French bread, so its price is 2f. The cinnamon raisin bread costs $2.50 more than the whole grain bread, so its price is (2f + 2.50). The total cost of the 3 loaves is $11.25, so we can set up the equations:

1. f + f + (f + 2.50) = 11.25
2. 2f + 2.50 = 11.25

Simplifying these equations gives us the correct choices A and D.

Answer 2

Option C.

Option E.

Step-by-step explanation:

Let be "f" the price of a loaf of French bread, "w" the price of the grain bread and "c" the price of the cinnamon raisin bread.

We know that Melissa paid twice as much for the whole grain bread as for the French bread. This can be represented with this equation:

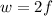`w=2f` [Equation 1]

Since she paid $2.50 more for the cinnamon raisin bread than for the whole grain bread, we can write this equation:

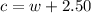`c=w+2.50` [Equation 2]

The total amount of money she spent was $11.25, then:

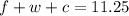`f+w+c=11.25` [Equation 3]

Now you must substitute the Equation 1 into the Equation 2:

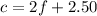`c=2f+2.50` [Equation 4]

Substituting the [Equation 1] and the [Equation 4] into the [Equation 3], you get:

`f+2f+(2f+2.50)=11.25`

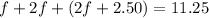
If you simplify the equation, you get:

`5f=8.75`