Question

A national newspaper reported that the state with the longest mean life span is Hawaii, where the population mean life span is 76 years. A random sample of 20 obituary notices in the Honolulu Advertizer gave the following information about life span (in years) of Honolulu residents.72 68 81 93 56 19 78 94 83 8477 69 85 97 75 71 86 47 66 27(i) Use a calculator with sample mean and standard deviation keys to find x and s. (Round your answers to two decimal places.)x-bar = ______ yrs = ______ yr

Answer 1

Mean = 71.4 years

Standard Deviation = 20.64 years

Explanation:

We are given the following data set:

72, 68, 81, 93, 56, 19, 78, 94, 83, 84, 77, 69, 85, 97, 75, 71, 86, 47, 66, 27

Formula:

`\text{Standard Deviation} = \sqrt{\displaystyle\frac{\sum (x_i -\bar{x})^2}{n-1}}`

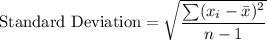
where
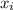`x_i` are data points,
`\bar{x}` is the mean and n is the number of observations.

`Mean = \displaystyle\frac{\text{Sum of all observations}}{\text{Total number of observation}}`

`Mean =\displaystyle(1428)/(20) = 71.4`

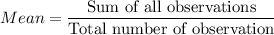
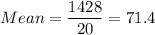
Sum of squares of differences =

0.36 + 11.56 + 92.16 + 466.56 + 237.16 + 2745.76 + 43.56 + 510.76 + 134.56 + 158.76 + 31.36 + 5.76, 184.96 + 655.36 + 12.96 + 0.16 + 213.16 + 595.36 + 29.16 + 1971.36 = 8100.8

`S.D = \sqrt{\displaystyle(8100.8)/(19)} = 20.64`

Mean = 71.4 years

Standard Deviation = 20.64 years