Question

According to a study conducted in one city, 35% of adults in the city have credit card debts more than $2.000. A simple random sample of n=250 adults is obtained from the city. Describe the sampling distribution of P^, the sample proportion of adults who have credit card debts of more than $2000.(Round to three decimal places when necessary.)Select from one of the 4 answers belowA. approximately- normal; \mu p=0.35, \sigma p=0.030B. approximately- normal; \mu p=0.35, \sigma p=0.001C. exactly- normal; \mu p=0.35, \sigma p=0.030D.Binomial; \mu p=87.5, \sigma p=7.542

Answer 1

Binomial; \mu p=87.5, \sigma p=7.542

Explanation:

• a distribution is said be a binomial distribution iff

1. The probability of success of that event( let it be p) is same for every trial
2. each trial should have 2 outcome : p or (1-p) i.e, success or failure only.
3. there are fixed number of trials (n)
4. the trials are independent

• here, the trials are obviously independent ( because, one person's debt doesn't influence the other person's)

• here n=250

• the probability of success(0.35) is same for every trial

(35/100=0.35 is the required p here)

• 
  `\mu_(p) =n*p=250*(35)/(100) =250*0.35=87.5`

[since, the formula for
`\mu _(p) =n*p` ]

• 
  `\sigma _(p) =√(n*(p)*(1-p)) = √(250*0.35*(1-0.35)) = 7.542 ( approximately)`

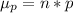[since, the formula for [tex]\sigma _{p} =\sqrt{n*(p)*(1-p)}

• therefore, it is Binomial; \mu p=87.5, \sigma p=7.542