Question

Evaluate the integral Integral ∫ from (1,2,3 ) to (5, 7,-2 ) y dx + x dy + 4 dz by finding parametric equations for the line segment from ​(1​,2​,3​) to ​(5​,7​,- 2​) and evaluating the line integral of of F = yi + x j+ 3k along the segment. Since F is conservative, the integral is independent of the path.

Answer 1

`\vec F(x,y,z)=y\,\vec\imath+x\,\vec\jmath+3\,\vec k`

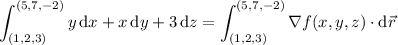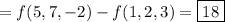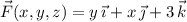
is conservative if there is a scalar function
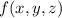`f(x,y,z)` such that
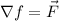`\\abla f=\vec F`. This would require

`(\partial f)/(\partial x)=y`

`(\partial f)/(\partial y)=x`

`(\partial f)/(\partial z)=3`

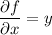
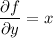
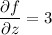
(or perhaps the last partial derivative should be 4 to match up with the integral?)

From these equations we find

`f(x,y,z)=xy+g(y,z)`

`(\partial f)/(\partial y)=x=x+(\partial g)/(\partial y)\implies(\partial g)/(\partial y)=0\implies g(y,z)=h(z)`

`f(x,y,z)=xy+h(z)`

`(\partial f)/(\partial z)=3=(\mathrm dh)/(\mathrm dz)\implies h(z)=3z+C`

`f(x,y,z)=xy+3z+C`

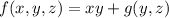
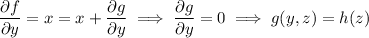
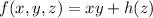
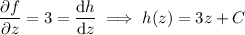
so
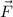`\vec F` is indeed conservative, and the gradient theorem (a.k.a. fundamental theorem of calculus for line integrals) applies. The value of the line integral depends only the endpoints:

`\displaystyle\int_((1,2,3))^((5,7,-2))y\,\mathrm dx+x\,\mathrm dy+3\,\mathrm dz=\int_((1,2,3))^((5,7,-2))\\abla f(x,y,z)\cdot\mathrm d\vec r`

`=f(5,7,-2)-f(1,2,3)=\boxed{18}`