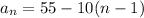Question

The fourth term of a sequence is 25.

A possible sequence is:

10, 15, 20, 25, 30, ...
a) Write down three possible sequence.

b) Write down the position to term rule for each of your
sequence in part a above

Answer 1

Answer below

Explanation:

Arithmetic Sequences

They can be identified because any term is obtained by adding or subtracting a fixed number to the previous term. That number is called the common difference.

The formula to calculate the nth term of an arithmetic sequence is:

`a_n=a_1+(n-1)r`

Where

an = nth term

a1 = first term

r = common difference

n = number of the term

Suppose we know the 4th term (n=4) of a sequence is 25:

`a_4=a_1+(4-1)r=25`

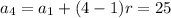
Simplifying:

a1 + 3r = 25

We can choose any combination of a1 and r to satisfy the equation above.

Solving for a1:

a1 = 25 - 3r

a)

Choosing r = 3:

a1 = 25 - 3*3 = 16

The sequence is:

16, 19, 22, 25, ...

And the term rule is:

`a_n=16+3(n-1)`

Choosing r=8

a1 = 25 - 3*8 = 1

The sequence is:

1, 9, 17, 25, ...

The term rule is:

`a_n=1+8(n-1)`

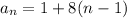
Choosing r=-10

a1 = 25 - 3*(-10) = 25 + 30 = 55

The sequence is:

55, 45, 35, 25, ...

The term rule is:

`a_n=55-10(n-1)`