Question

A spring with an 8-kg mass is kept stretched 0.4 m beyond its natural length by a force of 32 N. The spring starts at its equilibrium position and is given an initial velocity of 4 m/s. Find the position of the mass at any time t. (Use x for the displacement in meters from the equilibrium position.)

Answer 1

Step-by-step explanation:

mass, m = 8 kg

extension, y = 0.4 m

force, F = 32 N

maximum velocity, v = 4 m/s

maximum velocity , v = ω A

where, ω be the angular velocity and A be the amplitude

4 = ω x 0.4

ω = 10 rad/s

position

x = A Sin ωt

x = 0.4 Sin 10 t

Answer 2

x = 1.26 sin 3.16 t

Step-by-step explanation:

Assume that the general equation of the displacement given as

x = A sinω t

A=Amplitude ,t=time ,ω=natural frequency

We know that speed V

`V=(dx)/(dt)`

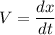
V= A ω cosωt

Maximum velocity

V(max)= Aω

Given that F= 32 N

F = K Δ

K=Spring constant

Δ = 0.4 m

32 =0.4 K

K = 80 N/m

We know that ω²m = K

8 ω² = 80

ω = 3.16 s⁻¹

Given that V(max)= Aω = 4 m/s

3.16 A = 4

A= 1.26 m

Therefore the general equation of displacement

x = 1.26 sin 3.16 t