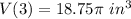Answer:
Part a)
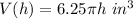
Part b)
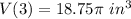 (see the explanation)
(see the explanation)
Explanation:
we know that
The volume of a cylinder is equal to
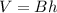
where
B is the area of the base of cylinder
h is the height of the cylinder
we have that
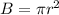
Part a) Write the function, V(h), that represents the volume of the cylinder
we have
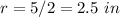 ----> the radius is half the diameter
----> the radius is half the diameter
substitute

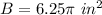
The volume is
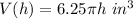
Part b) Find V(3) and tell what it represents
V(3) represent the volume of the cylinder with a height of 3 inches
so
For h=3 in
substitute