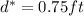Question

You’re in a mall and you need some money to buy a nose ring, but you’re broke. You decide to scoop some quarters out of the fountain. The water in the fountain is one foot deep. How far below the water do the quarters appear to be? The index of refraction of water is 4/3.

Answer 1

Answer: 0.75 ft

Step-by-step explanation:

This situation is due to Refraction, a phenomenon in which a wave (the light in this case) bends or changes its direction when passing through a medium with an index of refraction different from the other medium. In this context, the index of refraction is a number that describes how fast light propagates through a medium or material.

In addition, we have the following equation that states a relationship between the apparent depth
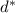`{d}^(*)` and the actual depth
`d`:

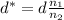`{d}^(*)=d\frac{{n}_(1)}{{n}_(2)}` (1)

Where:

`n_(1)=1` is the air's index of refraction

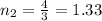`n_(2)=(4)/(3)=1.33` water's index of refraction.

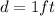`d=1 ft` is the actual depth of the quarters

Now. when
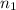`n_(1)` is smaller than
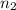`n_(2)` the apparent depth is smaller than the actual depth. And, when
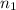`n_(1)` is greater than
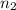`n_(2)` the apparent depth is greater than the actual depth.

Let's prove it:

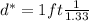`{d}^(*)=1 ft(1)/(1.33)` (2)

Finally we find the aparent depth of the quarters, which is smaller than the actual depth:

`{d}^(*)=0.75 ft`