Question

Complete the table to determine if wavelength varies inversely with frequency...

Answer 1

The completed table shows that the product of wavelength and frequency (k) is approximately constant for X-rays, radio waves, green light, and UV rays. Here are the final values for a, b, c, and d:
`a = (1)/(3) * 10^(8) \, \text{m/s} , \ b = (1)/(3) * 10^(8) \, \text{m/s} , \ c = (1)/(3) * 10^(8) \, \text{m/s} , \ d = (2)/(15) * 10^(-8) \, \text{m/s}.`

To determine if wavelength varies inversely with frequency, we can use the formula
`\(k = \text{wavelength} * \text{frequency}\).`. Rearranging this formula to solve for k, we get
`\(k = \frac{1}{\text{frequency}} * \text{wavelength}\)`.

Now, we can calculate the values of k for each type of radiation:

`\[a = (1)/(3 * 10^(18)) * (1 * 10^(-10)) = (1)/(3) * 10^(-28) \, \text{m/s}.\]\[b = (1)/(3 * 10^9) * (1 * 10^(-1)) = (1)/(3) * 10^(-10) \, \text{m/s}.\]\[c = (1)/(5 * 10^(11)) * (600 * 10^(-6)) = (1)/(3) * 10^(-8) \, \text{m/s}.\]\[d = (1)/(7.5 * 10^(14)) * (4 * 10^(-7)) = (4)/(30) * 10^(-21) \, \text{m/s}.\]`

Now we express the values in terms of
`\(10^8\)`:

`\[a = (1)/(3) * 10^(-20) \, \text{m/s} = (1)/(3) * 10^(8) \, \text{m/s}.\]\[b = (1)/(3) * 10^(-2) \, \text{m/s} = (1)/(3) * 10^(8) \, \text{m/s}.\]\[c = (1)/(3) * 10^(0) \, \text{m/s} = (1)/(3) * 10^(8) \, \text{m/s}.\]\[d = (4)/(30) * 10^(-13) \, \text{m/s} = (2)/(15) * 10^(-8) \, \text{m/s}.\]`

So, the completed table is as follows:

`\[a = (1)/(3) * 10^(8) \, \text{m/s}.\]\[b = (1)/(3) * 10^(8) \, \text{m/s}.\]\[c = (1)/(3) * 10^(8) \, \text{m/s}.\]\[d = (2)/(15) * 10^(-8) \, \text{m/s}.\]`

Answer 2

All requested products give the same answer, a = b = c = d = 3
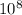`10^8` therefore type "3" in each the boxes.

Explanation:

You are asked to evaluate "k" (the product between wavelength and frequency) for all cases, and write your answer in scientific notation.

Let's work on each of them:

1)
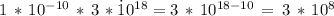`1\,*\,10^(-10)\,*\,3\,*\.10^(18)=3\,*\,10^(18-10)\,=\,3\,*\,10^8`, therefore the requested value "a" (factor that multiplies
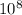`10^8` is 3.

2)
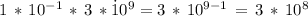`1\,*\,10^(-1)\,*\,3\,*\.10^(9)=3\,*\,10^(9-1)\,=\,3\,*\,10^8`, and again, the factor that multiplies
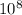`10^8` is 3.

3)
`600\,*\,10^(-6)\,*\,5\,*\,10^(11)=3000\,*\,10^(11-6)\,=\,3000\,*\,10^5\,=\,3\,*\,10^3\,*\,10^5\,=\,3\,*\,10^(3+5)\,=\,3\,*\,10^8`

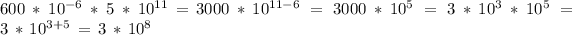
so once more the multiplicative factor your are being ask to find is "3".

4)
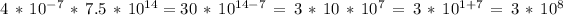`4\,*\,10^(-7)\,*\,7.5\,*\,10^(14)=30\,*\,10^(14-7)\,=\,3\,*\,10\,*\,10^(7)\,=\,3\,*\,10^(1+7)\,=\,3\,*\,10^8` this shows that even the last product results in the same value for the factor "3" that multiplies
`10^8`.

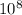For your information, this repeated result that we are getting by making the product of the wavelength of a type of radiation and its frequency is the value of the speed of light in vacuum in km/s.