Answer: He traveled 10 km/hr through bike and 5km/hr by jogging.
Explanation:
Let the speed of bike be 'x'.
Let the speed of his jogging be 'x-5'.
Distance covered = 10 miles
So the jog took 1 hour longer than the bike ride.
According to question, we get that
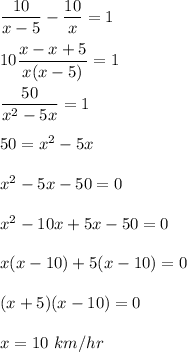
Hence, he traveled 10 km/hr through bike and 5km/hr by jogging.