Question

Solve the rational function of (check image) and check for extraneous solutions

Answer 1

Option A is correct.

i.e. x = 1, x = 0 is an extraneous solution.

Explanation:

Given the expression

`(5)/(x)=(4x+1)/(x^2)`

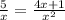
Solving the rational function

`(5)/(x)=(4x+1)/(x^2)`

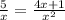
Apply fraction across multiply: if
`(a)/(b)=(c)/(d)\mathrm{\:then\:}a\cdot \:d=b\cdot \:c`

`5x^2=x\left(4x+1\right)`

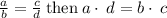
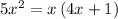
Subtract x(4x+1) from both sides

`5x^2-x\left(4x+1\right)=x\left(4x+1\right)-x\left(4x+1\right)`

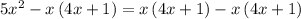
Simplify

`5x^2-x\left(4x+1\right)=0`

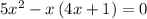
5x² - 4x² - x = 0

x² - x = 0

Factor x² - x = x(x-1)

so

x(x-1) = 0

Using the zero factor principle

if ab=0, then a=0 or b=0 (or both a=0 and b=0)

`x=0\quad \mathrm{or}\quad \:x-1=0`

Thus, the solution to the equation is:

`x=0,\:x=1`

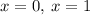
But, it is clear that if we substitute x = 0, the equation becomes undefined because we can not have the denominator to be 0.

In other words, the equation is undefined for x = 0

Thus, x = 0 is an extraneous solutions.

Therefore, option A is correct.

i.e. x = 1, x = 0 is an extraneous solution.