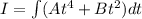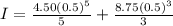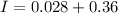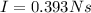Answer:
Part a)
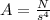
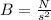
PART B)
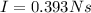
Step-by-step explanation:
PART A)
As we know that the force is given as
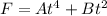
here we know that each term of the equation must have same dimensions
so we will have
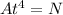
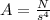
similarly for other term
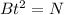
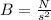
PART B)
Impulse given by the force is given as

now we have