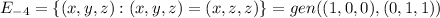Answer:
We have the matrix
![A=\left[\begin{array}{ccc}-4&-4&-4\\0&-8&-4\\0&8&4\end{array}\right]](https://img.qammunity.org/2020/formulas/mathematics/college/46sqt6k9926jutsfytaql4rke97r3zjfbs.png)
To find the eigenvalues of A we need find the zeros of the polynomial characteristic
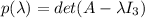
Then
![p(\lambda)=det(\left[\begin{array}{ccc}-4-\lambda&-4&-4\\0&-8-\lambda&-4\\0&8&4-\lambda\end{array}\right] )\\=(-4-\lambda)det(\left[\begin{array}{cc}-8-\lambda&-4\\8&4-\lambda\end{array}\right] )\\=(-4-\lambda)((-8-\lambda)(4-\lambda)+32)\\=-\lambda^3-8\lambda^2-16\lambda](https://img.qammunity.org/2020/formulas/mathematics/college/5u5ja4enkzjhcx2rgtch1n0sphl0ye0kj6.png)
Now, we fin the zeros of
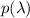 .
.
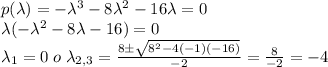
Then, the eigenvalues of A are
 of multiplicity 1 and
of multiplicity 1 and
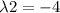 of multiplicity 2.
of multiplicity 2.
Let's find the eigenspaces of A. For
 :
:
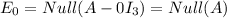 .Then, we use row operations to find the echelon form of the matrix
.Then, we use row operations to find the echelon form of the matrix
![A=\left[\begin{array}{ccc}-4&-4&-4\\0&-8&-4\\0&8&4\end{array}\right]\rightarrow\left[\begin{array}{ccc}-4&-4&-4\\0&-8&-4\\0&0&0\end{array}\right]](https://img.qammunity.org/2020/formulas/mathematics/college/29c36qqus4pkgl622uaiyfzdytu3pufdup.png)
We use backward substitution and we obtain
1.
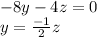
2.

Therefore,
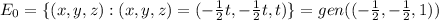
For
 :
:
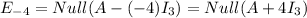 .Then, we use row operations to find the echelon form of the matrix
.Then, we use row operations to find the echelon form of the matrix
![A+4I_3=\left[\begin{array}{ccc}0&-4&-4\\0&-4&-4\\0&8&8\end{array}\right] \rightarrow\left[\begin{array}{ccc}0&-4&-4\\0&0&0\\0&0&0\end{array}\right]](https://img.qammunity.org/2020/formulas/mathematics/college/fa3ztr83xmyr7g44cpkk5b16g1oiwvlzac.png)
We use backward substitution and we obtain
1.
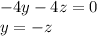
Then,