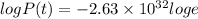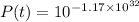Question

Tennis balls traveling at greater than 100 mph routinely bounce off tennis rackets. At some sufficiently high speed, however, the ball will break through the strings and keep going. The racket is a potential-energy barrier whose height is the energy of the slowest string-breaking ball. Suppose that a 100 g tennis ball traveling at 200 mph is just sufficient to break the 2.0-mm-thick strings. Estimate the probability that a 120 mph ball will tunnel through the racket without breaking the strings. Give your answer as a power of 10 rather than a power of e.

Answer 1

Probability of tunneling is
`10^{- 1.17* 10^(32)}`

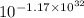
Solution:

As per the question:

Velocity of the tennis ball, v = 120 mph = 54 m/s

Mass of the tennis ball, m = 100 g = 0.1 kg

Thickness of the tennis ball, t = 2.0 mm =
`2.0* 10^(- 3)\ m`

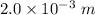
Max velocity of the tennis ball,
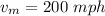`v_(m) = 200\ mph` = 89 m/s

Now,

The maximum kinetic energy of the tennis ball is given by:

`KE = (1)/(2)mv_(m)^(2) = (1)/(2)* 0.1* 89^(2) = 396.05\ J`

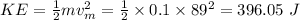
Kinetic energy of the tennis ball, KE' =
`(1)/(2)mv^(2) = 0.5* 0.1* 54^(2) = 154.8\ m/s`

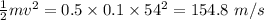
Now, the distance the ball can penetrate to is given by:

`\eta = \frac{\bar{h}}{√(2m(KE - KE'))}`

`\bar{h} = (h)/(2\pi) = (6.626* 10^(- 34))/(2\pi) = 1.0545* 10^(- 34)\ Js`

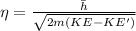
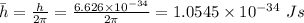
Thus

`\eta = (1.0545* 10^(- 34))/(√(2* 0.1(396.05 - 154.8)))`

`\eta = (1.0545* 10^(- 34))/(√(2* 0.1(396.05 - 154.8)))`

`\eta = 1.52* 10^(-35)\ m`

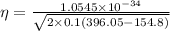
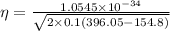
Now,

We can calculate the tunneling probability as:

`P(t) = e^{(- 2t)/(\eta)}`

`P(t) = e^{(- 2* 2.0* 10^(- 3))/(1.52* 10^(-35))} = e^{-2.63* 10^(32)}`

`P(t) = e^{-2.63* 10^(32)}`

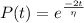
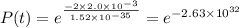
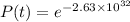
Taking log on both the sides:

`logP(t) = -2.63* 10^(32) loge`

`P(t) = 10^{- 1.17* 10^(32)}`