Answer:
(a)
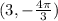
(b)

(c)
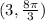
Explanation:
All polar coordinates of point (r, θ ) are
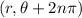 and
and
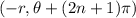
where, θ is in radian and n is an integer.
The given point is
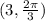 . So, all polar coordinates of point are
. So, all polar coordinates of point are
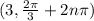 and
and
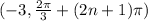
(a)
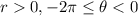
Substitute n=-1 in
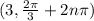 , to find the point for which
, to find the point for which
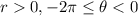 .
.
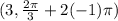
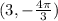
Therefore, the required point is
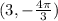 .
.
(b)
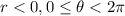
Substitute n=0 in
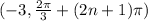 , to find the point for which
, to find the point for which
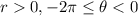 .
.
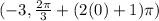
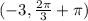

Therefore, the required point is
 .
.
(c)
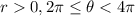
Substitute n=1 in
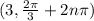 , to find the point for which
, to find the point for which
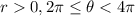 .
.
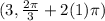
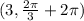
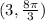
Therefore, the required point is
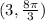 .
.