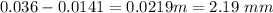Answer:
The distance between the bright fringes is 2.19 mm
Step-by-step explanation:
Distance of the screen from the slits, d = 1.9 m
Slit width, w = 0.11 mm =

Wavelength,
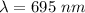
Wavelength,
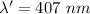
To calculate the distance between the second order bright fringe:
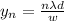
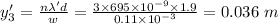
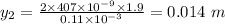
Distance, |x| =

|x| =