Answer:
The price of a sandwich is £2.5 and the price of a croissant is £2
Explanation:
Let s be the number of sandwiches and c be the number of croissants
Then according to given statements the equations will be:

Multiplying equation 1 by 2 and subtracting equation 2 from the new equation
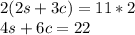
Now
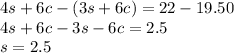
Putting s=2.5 in equation 1
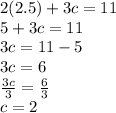
Hence,
The price of a sandwich is £2.5 and the price of a croissant is £2